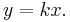Proportionality (mathematics)

y is directly proportional to x.

In mathematics, two quantities are said to be proportional if they vary in such a way that one of the quantities is a constant multiple of the other, or equivalently if they have a constant ratio.

Proportion also refers to the equality of two ratios.

Contents

Symbol

The mathematical symbol '∝' is used to indicate that two values are proportional. For example, A ∝ B.

In Unicode this is symbol U+221D.

Direct proportionality

Given two variables x and y, y is (directly) proportional to x (x and y vary directly, or x and y are in direct variation) if there is a non-zero constant k such that

y = kx.\,

The relation is often denoted

y \propto x

and the constant ratio

k = y/x\,

is called the proportionality constant or constant of proportionality.

Examples

Properties

Since

y = kx\,

is equivalent to

x = \left(\frac{1}{k}\right)y,

it follows that if y is proportional to x, with (nonzero) proportionality constant k, then x is also proportional to y with proportionality constant 1/k.

If y is proportional to x, then the graph of y as a function of x will be a straight line passing through the origin with the slope of the line equal to the constant of proportionality: it corresponds to linear growth.

Inverse proportionality

As noted in the definition above, two proportional variables are sometimes said to be directly proportional. This is done so as to contrast direct proportionality with inverse proportionality.

Two variables are inversely proportional (or varying inversely, or in inverse variation, or in inverse proportion or reciprocal proportion) if one of the variables is directly proportional with the multiplicative inverse (reciprocal) of the other, or equivalently if their product is a constant. It follows that the variable y is inversely proportional to the variable x if there exists a non-zero constant k such that

y = {k \over x}

The constant can be found by multiplying the original x variable and the original y variable.

Basically, the concept of inverse proportion means that as the absolute value or magnitude of one variable gets bigger, the absolute value or magnitude of another gets smaller, such that their product (the constant of proportionality) is always the same.

For example, the time taken for a journey is inversely proportional to the speed of travel; the time needed to dig a hole is (approximately) inversely proportional to the number of people digging.

The graph of two variables varying inversely on the Cartesian coordinate plane is a hyperbola. The product of the X and Y values of each point on the curve will equal the constant of proportionality (k). Since k can never equal zero, the graph will never cross either axis.

Hyperbolic coordinates

The concepts of direct and inverse proportion lead to the location of points in the Cartesian plane by hyperbolic coordinates; the two coordinates correspond to the constant of direct proportionality that locates a point on a ray and the constant of inverse proportionality that locates a point on a hyperbola.

Exponential and logarithmic proportionality

A variable y is exponentially proportional to a variable x, if y is directly proportional to the exponential function of x, that is if there exist non-zero constants k and a

y = k a^x.\,

Likewise, a variable y is logarithmically proportional to a variable x, if y is directly proportional to the logarithm of x, that is if there exist non-zero constants k and a

y = k \log_a (x).\,

Experimental determination

To determine experimentally whether two physical quantities are directly proportional, one performs several measurements and plots the resulting data points in a Cartesian coordinate system. If the points lie on or close to a straight line that passes through the origin (0, 0), then the two variables are probably proportional, with the proportionality constant given by the line's slope.

See also

Growth